HY8:AOP SS Gradations: Difference between revisions
No edit summary |
|||
| (5 intermediate revisions by 2 users not shown) | |||
| Line 5: | Line 5: | ||
==Reach Channel Gradations== | ==Reach Channel Gradations== | ||
You can enter several gradations across your stream. The first value you will need to define is how many gradations you wish to enter. Then in the first column of the table, you can change the name or leave the default name. You then define where stations between which the gradation was gathered and determined from. The cross section will use the gradation that is closest to it. | You can enter several gradations across your stream. The first value you will need to define is how many gradations you wish to enter. Then in the first column of the table, you can change the name or leave the default name. You then define where stations between which the gradation was gathered and determined from. The cross-section will use the gradation that is closest to it. | ||
You then define the gradation, by supplying the D5, D16, D50, D84, and D95 of the streambed. If you need some tools to determine the gradation, use the 'Rock/Sediment Calculator' from Hydraulic Toolbox, developed for FHWA by Aquaveo. This calculator allows you to enter a gradation by entering a Wolman Count, an image gradation (you enter a picture of riprap with a scale, and it will calculate a gradation), or a standard riprap. The standard riprap can be personalized by installing Hydraulic Toolbox and modifying the profiles. | You then define the gradation, by supplying the D5, D16, D50, D84, and D95 of the streambed. If you need some tools to determine the gradation, use the 'Rock/Sediment Calculator' from Hydraulic Toolbox, developed for FHWA by Aquaveo. This calculator allows you to enter a gradation by entering a Wolman Count, an image gradation (you enter a picture of riprap with a scale, and it will calculate a gradation), or a standard riprap. The standard riprap can be personalized by installing Hydraulic Toolbox and modifying the profiles. | ||
| Line 11: | Line 11: | ||
Only one gradation is allowed to be defined at any location. When the gradation is properly defined, the calculated D50 of the gradation will show in the final column. | Only one gradation is allowed to be defined at any location. When the gradation is properly defined, the calculated D50 of the gradation will show in the final column. | ||
Finally you can select method to compute the Manning's n value. When you make a change in the definition of one of the gradation or its station, HY-8 will auto-select a method. It is recommended that the user update the selected method to match the If there is a tie, HY-8 will select the method that provides an average value that is closest to 0.03. It is recommended that you review the selected method. To learn more about the methods and the required criteria of each method, see [http://www.fhwa.dot.gov/engineering/hydraulics/pubs/11008/ HEC-26] Appendix C. | Finally, you can select method to compute the Manning's n value. When you make a change in the definition of one of the gradation or its station, HY-8 will auto-select a method. It is recommended that the user update the selected method to match the If there is a tie, HY-8 will select the method that provides an average value that is closest to 0.03. It is recommended that you review the selected method. To learn more about the methods and the required criteria of each method, see [http://www.fhwa.dot.gov/engineering/hydraulics/pubs/11008/ HEC-26] Appendix C. | ||
Once the data | Once the data are entered, HY-8 will provide the number of calculations performed that met the criteria of the method selected and the average value of all the calculations given. These values are not used for any computations, but they are reported to the user to facilitate selecting a Manning's n computation method. The result of the calculation at each cross section with each flow is then reported in the final table of the spreadsheet. It is important to note that the average value of a method may be reasonable, but many of the individual calculations could still be very unreasonable. | ||
==Manning's n Methods== | ==Manning's n Methods== | ||
From HEC-26, Appendix C: | From [http://www.fhwa.dot.gov/engineering/hydraulics/pubs/11008/ HEC-26], Appendix C: | ||
An appropriate equation selection must consider the basis on which the equation was developed and how it might apply within a closed conduit. The Bathurst, Jarrett, and Mussetter equations tend to better represent n values on steeper channels or channels with larger roughness elements. Limerinos and Blodgett attempt to encompass a wider range of conditions. The Bathurst equation depends on channel top width for calculation of Manning’s n (See Kilgore and Cotton (2005) for details). However, in a closed conduit, top width does not monotonically increase with depth as it does in a natural channel. Therefore, the Bathurst equation would be problematic to apply within a culvert. | An appropriate equation selection must consider the basis on which the equation was developed and how it might apply within a closed conduit. The Bathurst, Jarrett, and Mussetter equations tend to better represent n values on steeper channels or channels with larger roughness elements. Limerinos and Blodgett attempt to encompass a wider range of conditions. The Bathurst equation depends on channel top width for calculation of Manning’s n (See Kilgore and Cotton (2005) for details). However, in a closed conduit, top width does not monotonically increase with depth as it does in a natural channel. Therefore, the Bathurst equation would be problematic to apply within a culvert. | ||
| Line 23: | Line 23: | ||
==Reach Stability== | ==Reach Stability== | ||
Finally, the user must make a stream stability assessment and then answer the Reach stability questions. Is the streambed in Dynamic Equilibrium? If not, does the channel stability support culvert design. If the answer to both questions is no, the stream needs to be stabilized before the aquatic organism passage can be determined. | Finally, the user must make a stream stability assessment and then answer the Reach stability questions. Is the streambed in Dynamic Equilibrium? If not, does the channel stability support culvert design. If the answer to both questions is no, the stream needs to be stabilized before the aquatic organism passage can be determined. | ||
This analysis must be performed by the engineer during a site visit. You find more information in [http://www.fhwa.dot.gov/engineering/hydraulics/pubs/11008/ HEC-26] on page 7-4, under the heading 'Step 3. Check for Dynamic Equilibrium' and continued through the heading 'Step 4. Analyze and Mitigate Channel Instability' on page 7-6. | |||
==Culvert Bed Gradations== | ==Culvert Bed Gradations== | ||
| Line 34: | Line 36: | ||
{{HY8Main}} | {{HY8Main}} | ||
[[Category:AOP| | [[Category:AOP|Grad]] | ||
[[Category:HY-8 Dialogs|AOP]] | |||
Latest revision as of 23:35, 28 February 2018
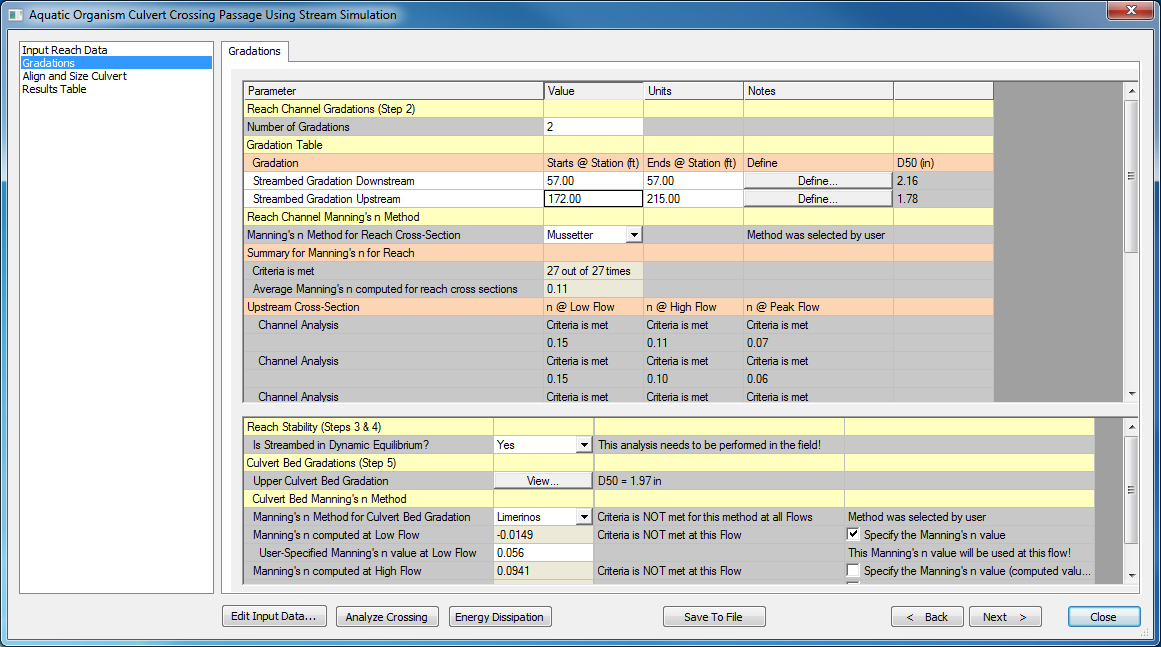
AOP Stream Simulation, Gradation Data
The gradations are used to determine the Manning's n value for the channel and culvert as well as the stability of the embedment within the culvert.
Reach Channel Gradations
You can enter several gradations across your stream. The first value you will need to define is how many gradations you wish to enter. Then in the first column of the table, you can change the name or leave the default name. You then define where stations between which the gradation was gathered and determined from. The cross-section will use the gradation that is closest to it.
You then define the gradation, by supplying the D5, D16, D50, D84, and D95 of the streambed. If you need some tools to determine the gradation, use the 'Rock/Sediment Calculator' from Hydraulic Toolbox, developed for FHWA by Aquaveo. This calculator allows you to enter a gradation by entering a Wolman Count, an image gradation (you enter a picture of riprap with a scale, and it will calculate a gradation), or a standard riprap. The standard riprap can be personalized by installing Hydraulic Toolbox and modifying the profiles.
Only one gradation is allowed to be defined at any location. When the gradation is properly defined, the calculated D50 of the gradation will show in the final column.
Finally, you can select method to compute the Manning's n value. When you make a change in the definition of one of the gradation or its station, HY-8 will auto-select a method. It is recommended that the user update the selected method to match the If there is a tie, HY-8 will select the method that provides an average value that is closest to 0.03. It is recommended that you review the selected method. To learn more about the methods and the required criteria of each method, see HEC-26 Appendix C.
Once the data are entered, HY-8 will provide the number of calculations performed that met the criteria of the method selected and the average value of all the calculations given. These values are not used for any computations, but they are reported to the user to facilitate selecting a Manning's n computation method. The result of the calculation at each cross section with each flow is then reported in the final table of the spreadsheet. It is important to note that the average value of a method may be reasonable, but many of the individual calculations could still be very unreasonable.
Manning's n Methods
From HEC-26, Appendix C:
An appropriate equation selection must consider the basis on which the equation was developed and how it might apply within a closed conduit. The Bathurst, Jarrett, and Mussetter equations tend to better represent n values on steeper channels or channels with larger roughness elements. Limerinos and Blodgett attempt to encompass a wider range of conditions. The Bathurst equation depends on channel top width for calculation of Manning’s n (See Kilgore and Cotton (2005) for details). However, in a closed conduit, top width does not monotonically increase with depth as it does in a natural channel. Therefore, the Bathurst equation would be problematic to apply within a culvert.
Reach Stability
Finally, the user must make a stream stability assessment and then answer the Reach stability questions. Is the streambed in Dynamic Equilibrium? If not, does the channel stability support culvert design. If the answer to both questions is no, the stream needs to be stabilized before the aquatic organism passage can be determined.
This analysis must be performed by the engineer during a site visit. You find more information in HEC-26 on page 7-4, under the heading 'Step 3. Check for Dynamic Equilibrium' and continued through the heading 'Step 4. Analyze and Mitigate Channel Instability' on page 7-6.
Culvert Bed Gradations
The culvert may have two gradations: an upper layer and a lower layer. If only one layer is needed, the upper layer will be used. The upper Layer is computed by averaging all of the streambed gradations. When the streambed gradations are properly defined, the calculated D50 of the gradation will show in the final column of the upper culvert bed gradation. HY-8 will determine if the lower layer is needed and determine the size later.
Then, you can select method to compute the Manning's n value. When you make a change in the definition of one of the gradation, HY-8 will choose the method that has the most criteria that is met. If there is a tie, HY-8 will select the method that provides an average value that is closest to 0.03. It is recommended that you review the selected method. To learn more about the methods and the required criteria of each method, see HEC-26 Appendix C.
The final three rows show the results of the computations on the culvert bed.